Example 4

We chose this identity as an example because it appears in a lot of trigonometry books, and it is a good problem.

Just as usual, we start from left to right. Let's see what are the differences. This identity has all three differences. We can tell you there are several ways to prove this problem. First let's see the easiest way.

First we want to reduce the differences of operations. Arrange the four terms on the left into two groups. Try to make them become products. The first two terms is actually , by changing around . The last 2 terms on the left side is , which can also be converted to . Now we get:

When we look at the right side, we see that we already got something we need at the left side, which is . The operation on the right is multiplication. So we factor out, and get:

By using our compare strategy, we can see that if we pull out from right side, which is , then because both sides are equal, should equal to . If we use the Sum-to-Product formulas to reduce the difference of operations, we have:

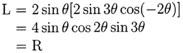
The identity has been proven.

The first way is the easiest and most natural way. But, by using more than one way to prove an identity, you can increase your problem solving skill tremendously. It can also help to "span" your brain faster.