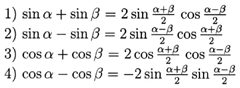
Notice--cosine sums have all cosine product or all sine product answers and sine sums have the mixed products of sine and cosine. When sums go to products the angles and become and . Note the distribution of angles and functions, and what formula has coefficient -2.

In formulas 1 and 2, be aware the order of functions in the products: cosine is behind sine. And the order of angles, sine plus sine gives the first factor and the second is . sine minus sine makes the first factor and the second .

In formulas 3 and 4, the sum of cosines of and become the product of cosines of and . The differences of cosines of and become the product of sines of and . Note in and have the same order, and the coefficient is negative 2, which is different from other three formulas.

We hope you can memorize all these formulas effectively, because they are really important. After you think you know all of them then go to Understanding the Formulas section to learn more about them. Or if you need more information about the basic trigonometric formulas, then come here for more information. Good luck.