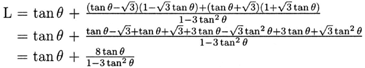[Index]

|
Example 3
![]()
We don't know if you already noticed that this is a hard one. Before
we do anything else, let's find out what are the differences between
the two sides. The angles and operations are different. Let's
look at it closely. The right side is ![]() .
If you did read our Double
& Triple Angle formulas in Memorizing
the formulas section, you should know it equals to
.
If you did read our Double
& Triple Angle formulas in Memorizing
the formulas section, you should know it equals to ![]() .
Believe it or not, you need to memorize it, or you will be in
trouble, at least in this problem. Now the right side looks like
this:
.
Believe it or not, you need to memorize it, or you will be in
trouble, at least in this problem. Now the right side looks like
this:
![]()
We will not say we can't, but we think it's not a good idea to prove it from the right side to the left side.
Once we know the right side, we need to compare both sides again.
Now, we see that they have two differences. It didn't change a
lot, but, it's very easy to change ![]() to
to ![]() and
and ![]() ,
and
,
and ![]() is special angle, the tangent of which could be changed to a special
number. So we use Sum formulas
to do this.
is special angle, the tangent of which could be changed to a special
number. So we use Sum formulas
to do this.
![]()
Now, we want to change the values of functions of special angles
to special numbers, so we leave ![]() there.
there.
![]()
We know that the right side is multiplication, so we want to change the operations of left side to multiplication too. If we simplify the left side, we get: