Example 1

We see that the functions of this identity are different, as well as the operations and angles. Sometimes we should reduce the differences of angles first, but this is not always. The big difference between both sides of this identity is difference of operations. The left side has addition and division, the right side has multiplication. What we should do is combine the left side to one term as multiplication, which will reduce the difference of operations. After we combine the left side we have:

Very natural, we need to continue simplifying it.

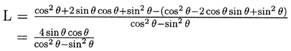
As we know, the angle of the right side is . So the next step is to change angles on the left side to . By using Double Angle formulas, and . We get:

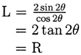
The identity has been proven.

At the last step, without thinking we will change to , but actually, we are reducing the differences of functions.

We know that some people might think without using our idea, they still can prove this identity. Yes, it's true, but most of them can't explain how they solve them. In this Examples section, when you see some hard problem, you will know how our idea helps.