[Index]
Introduction
Essence
Memorizing
Understanding
Summarizing
Examples
Example 1
Example 2
Example 3
Example 4
Example 5
Example 6
Example 7
Example 8
General ideas
Exercises
Final thoughts
|
Example 8--Page 4
All right, here is the second way. To understand how the second way works, you have to read our Summarize the Formulas section. If you haven't, please go back and take a look at it.
There is one formula in our Summarize the Formulas section:
where 
satisfies 
,
or 
(if
a =
0). The identity 
has 
,
which reminds us that this formula might be able to help us to
solve the problem.
By using this formula, we get:
| From (1) |  |
(3) |
| From (2) |  |
(4) |
When we look at it, we are very happy, because we already got ,
and at the same time, we have c.
You might want to square the both sides of those equations. Yes,
we will do it, but it's not the right time yet, because we know
that we want to have 
,
so we will use the Sum to Product
formulas. To do that, we add equations (3) and (4).
| (3) + (4) |  |
 |
Dividing 2 from both sides, we have:
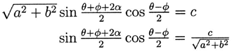
Now we are almost there. Next we will just square both sides and we have:
 |
(5) |
Again, using our compare strategy, we believe that 
must be equal to 1, so that the identity will be true. We claim
that:
 |
(6) |
