|
Light version
|
Essence
Memorizing
Understanding
Summarizing
Examples
Example 1
Example 2
Example 3
Example 4
Example 5
Example 6
Example 7
Example 8
General ideas
Final thoughts
Example 6
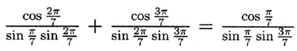
Actually this is a kind of conditional identity,
because it has the actual angles. If the angles change, this identity
may not be true. In some identities, it doesn't matter what are
the angles. You should know that none of the angles in this problem
are special angles. They are not like 
,
,
,
and 
,
so we can't convert their functions to special numbers. If there
are conditions for a given identity, we will need to use them
when we prove the identity, or it's none sense to have conditions.
We will use the conditions--the angles 
,
,
etc, to prove this identity.
After comparing the two sides we see that the big difference is the difference of operations. To reduce it, we will combine the terms on the left.
By comparing what we get with the right side, we believe that 
,
because we already get 
.
If we can prove that, then the problem is solved. So we separate
the left to two factors.
