|
Light version
|
Essence
Memorizing
Understanding
Summarizing
Examples
Example 1
Example 2
Example 3
Example 4
Example 5
Example 6
Example 7
Example 8
General ideas
Final thoughts
Example 2
The difference of angles is sharp. The right side has no 
,
so first we want to cancel
in left side.
If you read our Memorizing the
formulas section, this problem should not be a hard one for
you because we included three Triple
Angle formulas there. Two of these we will use here. Namely,
and 
.
We see we can factor 
from 
,
and 
from 
.
Thus we can simplify 
and 
.
By using the Triple Angle formulas we get:
Being very straight forward, we want to factor out
and ,
at the top of the two fractions respectively, and cancel it with
the bottom.
Then combine the like terms, we have
We know 
can help us to cancel ,
so we factor out -4, we get,
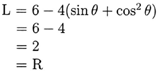
The identity has been proven.
It's not hard right? Without knowing our idea you can do it too, but can you give another proof?
